
It is used in Analysis of Beams and Columns.It is used in calculating Bending Stresses.It plays a major role in selecting and sizing the type of cross-sections to be used as flexural members in a structure.It is a cross-sectional property that can be used to predict the resistance of beams to bending and deflection.From the perpendicular axis theorem, J can be calculated as the sum of I x and I y.
While I represents resistance to bending, J represents resistance to twisting about the reference axis. Similar to I, Polar moment of inertia (J) represents the resistance of the shape of the cross-section. Unit of I is units 4 i.e., mm 4, in 4, m 4. Where x = perpendicular distance considered from Y-axis. As the reference axis is considered as X-axis, the moment of inertia thus calculated is.
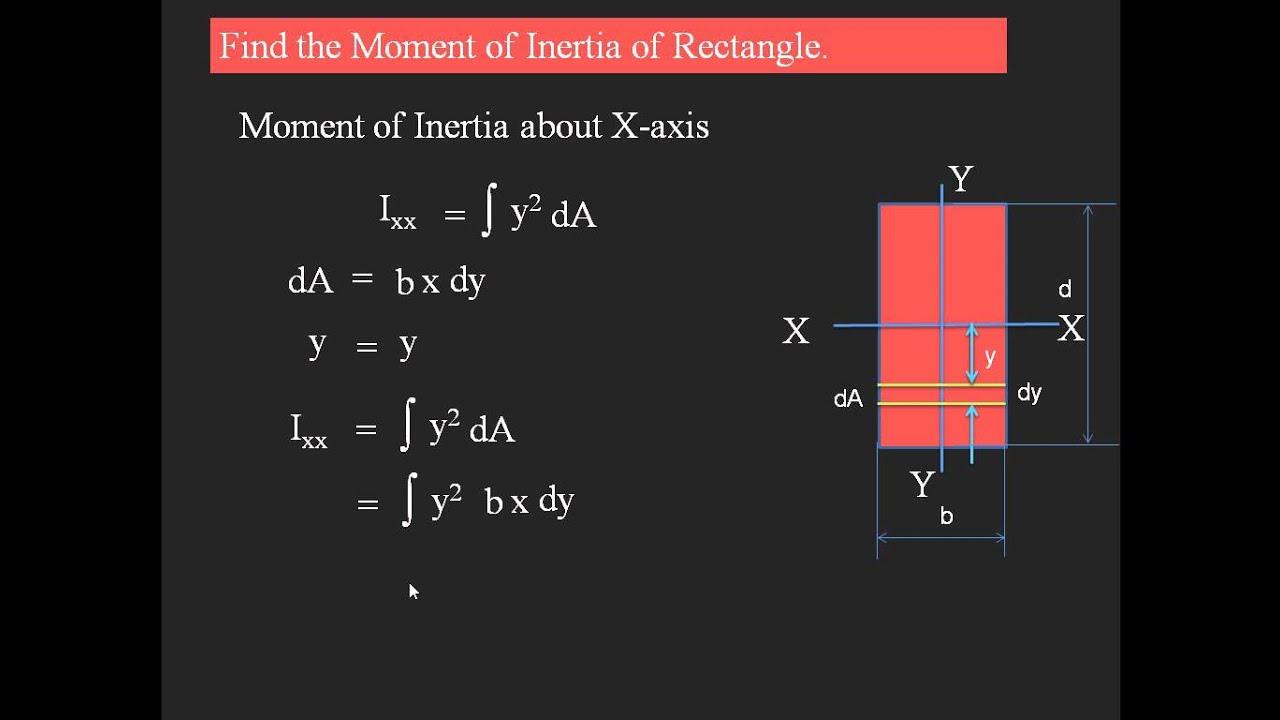
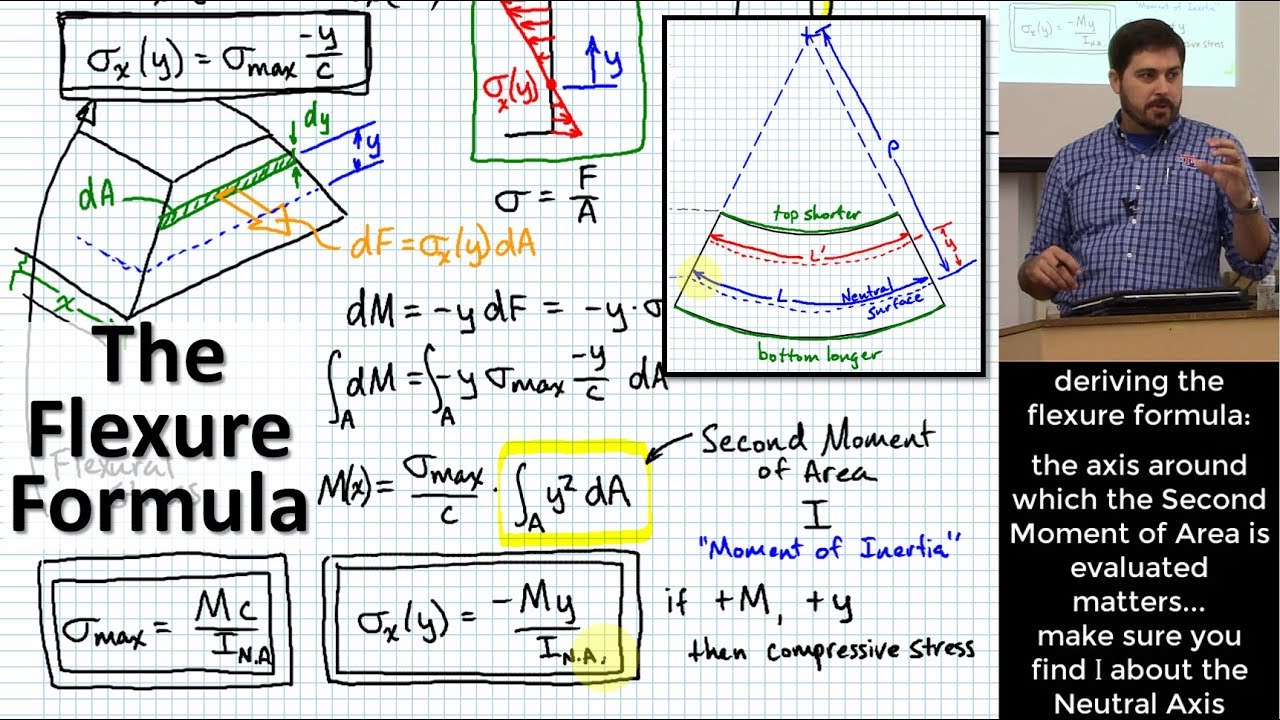
Where dA = area in consideration, y = perpendicular distance taken from X-axis. The following are the generalised formula for area moment of inertia. The perpendicular distance depends on the axis of bending. Second moment of area = (area) x (perpendicular distance) 2. It can be calculated by taking moment about the area twice i.e., Now that we have understood how a distributed area influences the value of I, we shall see about how exactly area moment of inertia of a section is calculated.Īrea moment of inertia is also known as the second moment of an area for a reason. HOW IS AREA MOMENT OF INERTIA CALCULATED? And the reason is it has its area distributed away from the centroidal axis. This is the major reason why sections shaped like an “I” is preferred over the rectangular cross-section.Īn I – section has a larger area concentration away from the centroidal axis compared to a rectangular cross-section of the same area as shown.įrom the above image, it can be easily understood that an I section has a higher value of area moment of inertia compared to the rectangular section of the same cross-sectional area. Thus, more the distance of the area from the bending axis higher is its area moment of inertia. Hence the beam 1 has a higher Area moment of inertia. This is because the beam 1 has a higher area moment of inertia about the bending axis compared to the beam 2.Īs you can see the area of the beam cross-section 1 is concentrated more away from the bending axis than in the beam 2. It can be seen that bending is low in case 1 compared to case 2. The only thing that changes is its geometry. Also, same beam, same area, same Young’s Modulus. In the picture shown, the cross-section of the plank is the same in both cases. It can be explained by a simple illustration. It is completely independent of the mass and other mechanical properties of the body. It can be defined as the resistance offered by a cross-sectional area to bending. Like, Young’s modulus is a stiffness due to material it is made of. Quick Answer: It is just a name that we gave to stiffness due to the shape of an element. So what is this “I” which is used all over in structural application? It is the Area Moment of Inertia or Second Moment of Area. The moment of inertia represented by “I” and used in deflection calculations of a beam, buckling load calculations of a column and in many other places in structural engineering has nothing related to the terms Mass or Inertia. Is this the parameter which is widely used in structural engineering as “ I“? The answer is NO! But what if the body is in a rotating motion? The resistance offered to the angular acceleration by a rotating body is called Moment of inertia (or) Mass moment of inertia. Now, this can be said for a body in a linear motion. Inertia is a property of a body under which it continues to be in a state of rest or uniform motion until it is acted upon by an external force. HOW IS AREA MOMENT OF INERTIA CALCULATED?.Double integration of the above equation leads to computing the deflection of the beam, and in turn, the bending stiffness of the beam.īending stiffness in beams is also known as Flexural rigidity. The bending stiffness ( K is the distance along the beam.


 0 kommentar(er)
0 kommentar(er)
